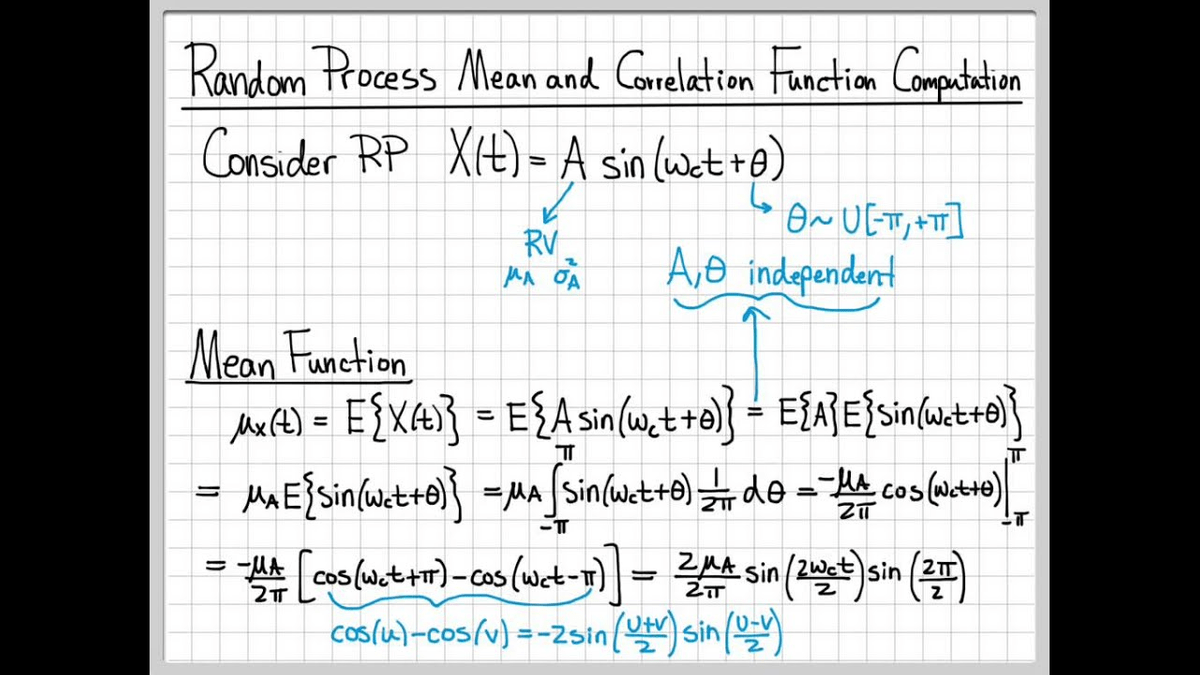
It is as if T_(i-1) captures all the information associated with values older than itself.īut what if this assumption were not true? What if the variance in T_(i-1) is not able to explain all of the variance contained within T_(i-2)? In that case, the above equation will not be able to feed this unexplained portion of the variance from T_(i-2) into T_i, causing the forecast for T_i to go off the mark.įortunately it’s easy to fix this problem adding a term to the above equation as follows: The key assumption behind this simple equation is that the variance in T_(i-1) is able to explain all the variance expressed by all values that are older than T_(i-1) in the time series. Beta1 tells us the rate at which T_i changes w.r.t.
It also specifies what will be the forecast for T_i if the value at the previous time step T_(i-1) happens to be zero. Beta0 is the Y-intercept of the model and it applies a constant amount of bias to the forecast. Here T_i is the value that is forecast by the equation at the ith time step.